Table of Contents
Narrow, crooked, self-avoiding plane-filling trails
On this page we see plane-filling trails visualizing the narrow and crooked, self-avoiding Fractal Pinwheel, Harter-Heighway Dragon, Ventrella's Family, and Gosper Stairway curves.
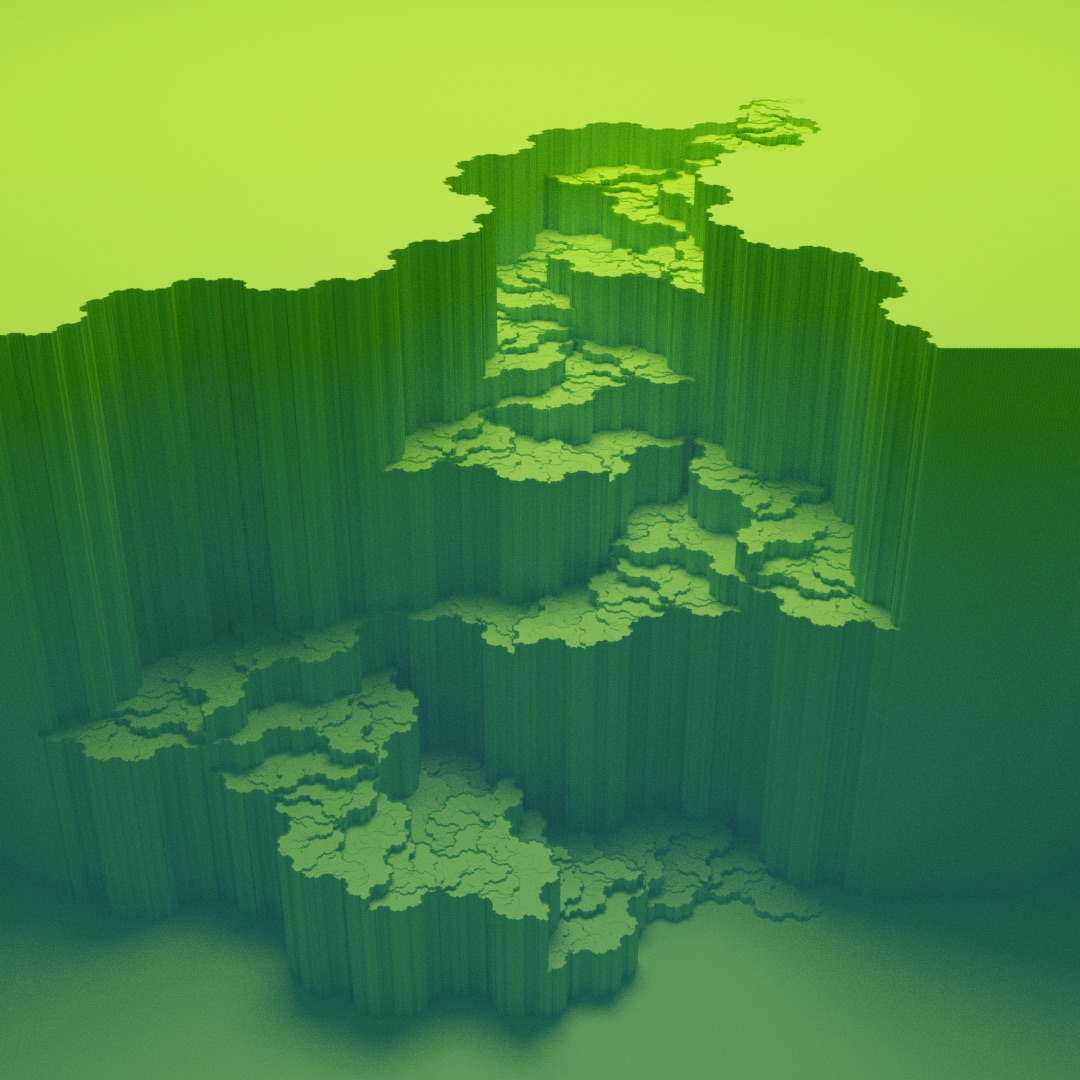
Fractal Pinwheel
Definition: (0,1,1,-1)(1,0,1,1)(0,1,-1,-1)(1,0,-1,1)(0,-1,-1,-1)
I found this curve in Ventrella's book1). The underlying tessellation is described by Bandt et al.2). A peculiar property of this curve is that, similar to the Pinwheel Triangle Curve and unlike almost all other curves on this website, the curve cannot be understood as the limit of approximating curves on a regular grid.
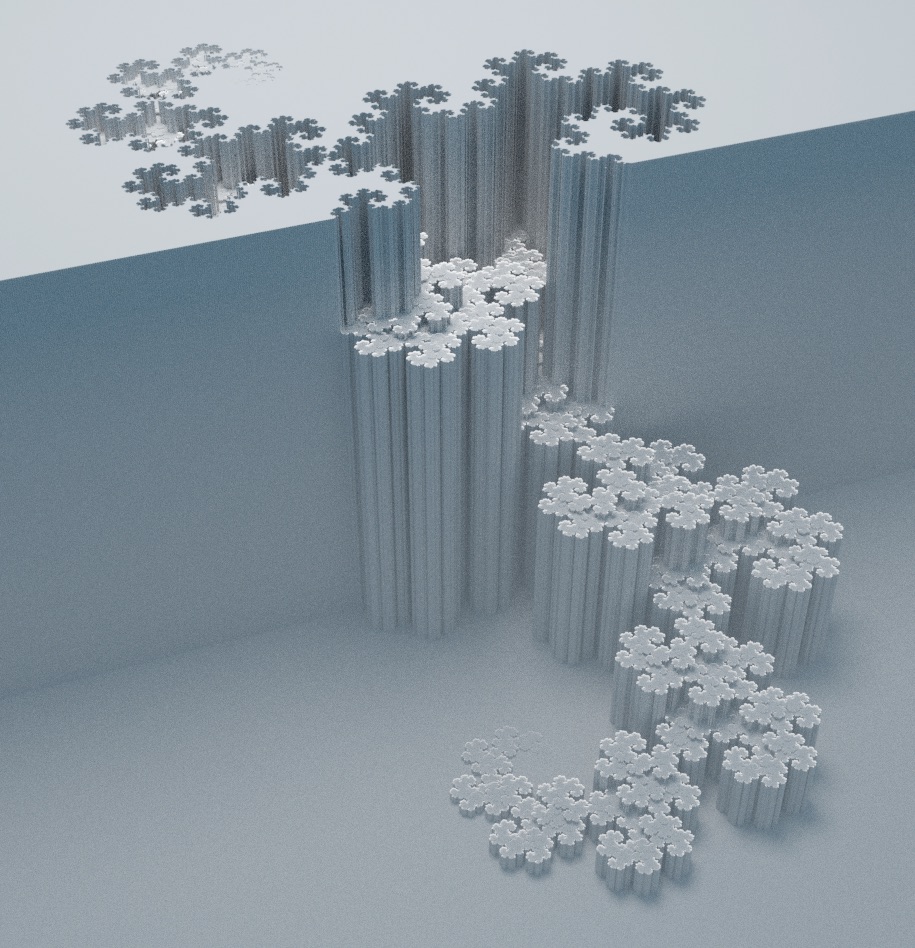
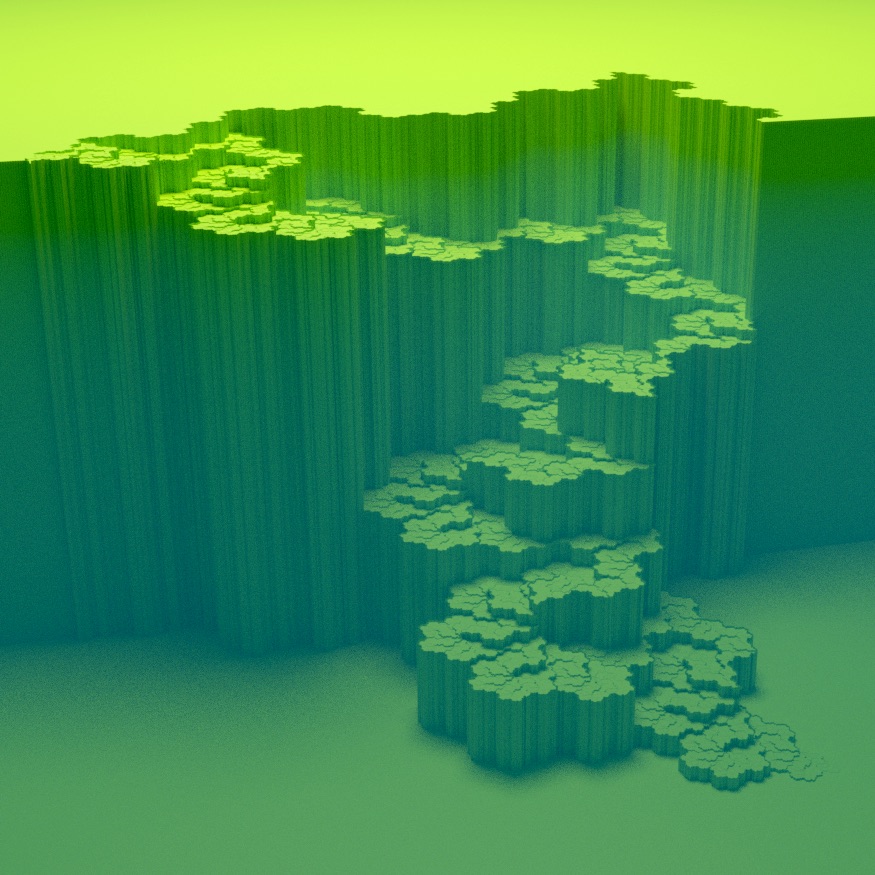
Harter-Heighway Dragon
Definition: (1,0,1,1)(0,1,-1,-1)
The well-known Harter-Heighway Dragon curve is one of the plane-filling curves that are simplest to define. In the plane-filling trail model, I hide almost half in the curve in the background material, but we know what it looks because of the first half. In return for hiding half of the curve in the background, we get a crisp boundary of the curve's image cut out in the upper plain.
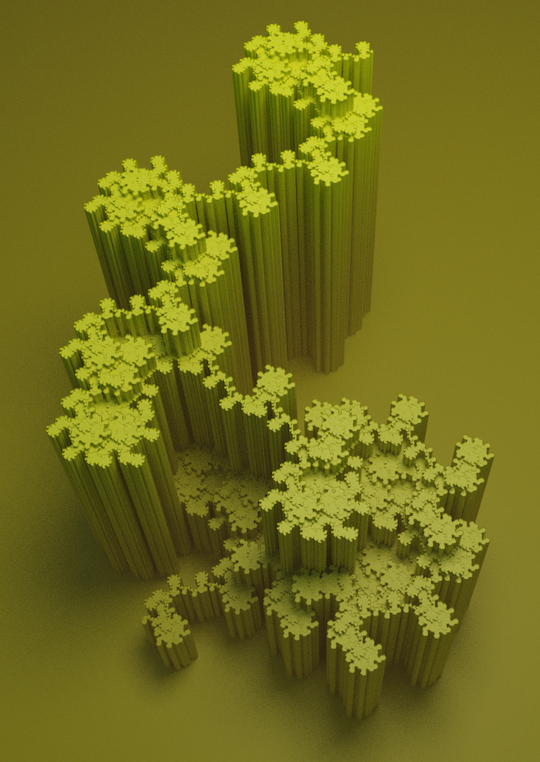
Ventrella's Family
Definition: Δ(1,0,0,-1,-1)(0,1,0,-1,-1)(1,0,0,-1,-1)(0,0,1,-1,-1)(0,0,1,-1,-1)(1,0,0,-1,-1)(1,0,0,-1,-1)
I found this curve in Ventrella's book3). I call it Ventrella's Family because it looks like a parent with two big children, and many smaller ones.
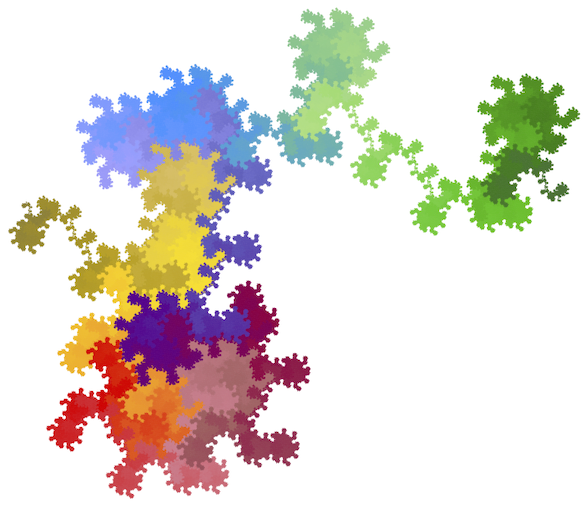
Gosper Stairway
Definition: Δ(1,0,0,1,1)(0,-1,0,1,1)(1,0,0,1,1)(-1,0,0,1,1)(0,1,0,1,1)(0,0,-1,1,1)(1,0,0,1,1)
This curve fills a shape that is like a triangle whose edges are replaced by the same fractal curves that make up the boundary of a Gosper island.